Tutorial 1

Tutorial 1: Functions, Derivatives & Engineering Applications

Evaluate the limit for the following if it exists:
a. $\displaystyle \lim _{x \rightarrow 5} \frac{x^{2}-25}{x^{2}+x-30}$
Solution
$\begin{aligned} \lim _{x \rightarrow 5} \frac{x^{2}-25}{x^{2}+x-30} &=\lim _{x \rightarrow 5} \frac{(x-5)(x+5)}{(x-5)(x+6)}\\ &=\lim _{x \rightarrow 5} \frac{(x+5)}{(x+6)}\\ &=\frac{(5+5)}{(5+6)}\\ &=\frac{10}{11} \end{aligned}$
b. $\displaystyle \lim _{x \rightarrow 9} \frac{\sqrt{x}-3}{x-9}$
Solution
$\begin{aligned} \lim _{x \rightarrow 9} \frac{\sqrt{x}-3}{x-9} &=\lim _{x \rightarrow 9} \frac{(\sqrt{x}-3)(\sqrt{x}+3)}{x-9(\sqrt{x}+3)}\\ &=\lim _{x \rightarrow 9} \frac{(x-9)}{(x-9)(\sqrt{x}+3)}\\ &=\lim _{x \rightarrow 9} \frac{1}{(\sqrt{x}+3)}\\ &=\frac{1}{(\sqrt{9}+3)}\\ &=\frac{1}{6} \end{aligned}$
c. $\displaystyle \lim _{x \rightarrow 0} \frac{x}{3-\sqrt{x+9}}$
Solution
$\begin{aligned} \lim _{x \rightarrow 0} \frac{x}{3-\sqrt{x+9}} &=\lim _{x \rightarrow 0} \frac{x}{(3-\sqrt{x+9)}} \frac{(3+\sqrt{x+9})}{(3+\sqrt{x+9)}}\\ &=\lim _{x \rightarrow 0} \frac{x(3+\sqrt{x+9})}{9-(x+9)}\\ &=\lim _{x \rightarrow 0} \frac{x(3+\sqrt{x+9})}{-x}\\ &=\lim _{x \rightarrow 0} \frac{3+\sqrt{x+9}}{-1}\\ &=-6 \end{aligned}$

Find the derivative of $\log(4+\cos x)$ .
Solution
$\begin{aligned} D(\log x) &=\frac{1}{x} \log e=\frac{1}{x \ln 10} \\ D[\log (4+\cos x)] &=\frac{1}{4+\cos x}(\log e) D(4+\cos x)\\ &=\frac{1}{4+\cos x}(\log e)(-\sin x)\\ &=\frac{-(\log e)(\sin x)}{4+\cos x} \end{aligned}$

Find $\frac{dy}{dx}$ for $\cos(x^2)=xe^y$ .
Solution
$\begin{aligned} \cos \left(x^{2}\right) &=x e^{y} \\ -\sin \left(x^{2}\right) \cdot 2 x &=x e^{y} \frac{d y}{d x}+e^{y}\\ x e^{y} \frac{d y}{d x} &=-2 x \sin \left(x^{2}\right)-e^{y} \\ \frac{d y}{d x} &=\frac{-2 x \sin \left(x^{2}\right)-e^{y}}{x e^{y}} \end{aligned}$
Find $\frac{dy}{dx}$ for $x^3y^3-2y=x$ .
Solution
$\begin{aligned} x^{3}\left[3 y^{2}\left(\frac{d y}{d x}\right)\right]+y^{3}\left[3 x^{2}\right]-2\left(\frac{d y}{d x}\right)&=1 \\ 3 x^{3} y^{2}\left(\frac{d y}{d x}\right)+3 x^{2} y^{3}-2\left(\frac{d y}{d x}\right)&=1 \\ 3 x^{3} y^{2}\left(\frac{d y}{d x}\right)-2\left(\frac{d y}{d x}\right)&=1-3 x^{2} y^{3} \\ \frac{d y}{d x}&=\frac{1-3 x^{2} y^{3}}{3 x^{3} y^{2}-2} \end{aligned}$
A curve in the plane is defined parametrically by the equations $x=7\ln(t)$ and $y=\sqrt{1-4t}$ . Find $\frac{dy}{dx}$ .
Solution
$\begin{aligned} \frac{d y}{d x}&=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}\\ &=\frac{\frac{d}{d t}(\sqrt{1-4 t})}{\frac{d}{d t}(7 \ln (t))}\\ &=\frac{\frac{1}{2}(1-4 t)^{-\frac{1}{2}} \cdot(-4)}{\frac{7}{t}}\\ &=-\frac{2 t}{7 \sqrt{1-4 t}} \end{aligned}$
A curve in the plane is defined parametrically by the equations $x=t^2-1$ and $y=2e^t$ . Find $\frac{dy}{dt}$ .
Solution
$\begin{aligned} \frac{d y}{d x}&=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}\\ &=\frac{\frac{d}{d t}\left(2 e^{t}\right)}{\frac{d}{d t}\left(t^{2}-1\right)}\\ &=\frac{2 e^{t}}{2 t}=\frac{e^{t}}{t} \end{aligned}$
Find $y'$ for each of the following:
a. $x^2\tan(y)+y^{10}\sec(x)=2x$
Solution
$\begin{aligned} 2 x \tan (y)+x^{2} \sec ^{2}(y) y^{\prime}&+10 y^{9} y^{\prime} \sec (x)+y^{10} \sec (x) \tan (x)=2 \\ \left(x^{2} \sec ^{2}(y)+10 y^{9} \sec (x)\right) y^{\prime}&=2-y^{10} \sec (x) \tan (x)-2 x \tan (y) \\ y^{\prime}&=\frac{2-y^{10} \sec (x) \tan (x)-2 x \tan (y)}{x^{2} \sec ^{2}(y)+10 y^{9} \sec (x)} \end{aligned}$
b. $x^3y^5+3x=8y^3+1$
Solution
$\begin{aligned} 3 x^{2} y^{5}+5 x^{3} y^{4} y^{\prime}+3&=24 y^{2} y^{\prime} \\ 3 x^{2} y^{5}+3&=24 y^{2} y^{\prime}-5 x^{3} y^{4} y^{\prime} \\ y^{\prime}&=\frac{3 x^{2} y^{5}+3}{24 y^{2}-5 x^{3} y^{4}} \end{aligned}$
c. $e^{2x+3y}=x^2-\ln(xy^3)$
Solution
$\begin{aligned} 2 e^{2 x+3 y}+3 y^{\prime} e^{2 x+3 y}&=2 x-\frac{y^{3}}{x y^{3}}-\frac{3 x y^{2} y^{\prime}}{x y^{3}} \\ 2 e^{2 x+3 y}+3 y^{\prime} e^{2 x+3 y}&=2 x-\frac{1}{x}-\frac{3 y^{\prime}}{y} \\ \left(3 e^{2 x+3 y}+3 y^{-1}\right) y^{\prime}&=2 x-x^{-1}-2 e^{2 x+3 y} \\ y^{\prime}&=\frac{2 x-x^{-1}-2 e^{2 x+3 y}}{3 e^{2 x+3 y}+3 y^{-1}} \end{aligned}$
Solve for $y'$ if $y=\ln(\cos x^2)$ .
Solution
Let $u=\cos x^{2}$ , then $\frac{d u}{d x}=\left(-\sin x^{2}\right)(2 x)$ ,
$y=\ln u, \text { then } \frac{d y}{d u}=\frac{1}{u}$
$\begin{aligned} \frac{d y}{d x}&=\left(\frac{1}{u}\right)\left(-\sin x^{2}\right)(2 x)\\ &=-\frac{2 x \sin x^{2}}{\cos x^{2}}\\ &=-2 x \tan x^{2} \end{aligned}$
Find $y'$ for $10e^{2xy}=e^{15y}+e^{13x}$ .
Solution
$\begin{aligned} 10 e^{2 x y}&=e^{15 y}+e^{13 x}\\ 10 e^{2 x y}\left(2 x y^{\prime}+2 y\right)&=e^{15 y}\left(15 y^{\prime}\right)+e^{13 x}(13)\\ 10 e^{2 x y}\left(2 x y^{\prime}+2 y\right)&=15 y^{\prime} e^{15 y}+13 e^{13 x} \\ \left(20 x e^{2 x y}-15 e^{15 y}\right) y^{\prime}&=13 e^{13 x}-20 y e^{2 x y} \\ y^{\prime}&=\frac{13 e^{13 x}-20 y e^{2 x y}}{20 x e^{2 x y}-15 e^{15 y}} \end{aligned}$
Solve $f'(x)$ if $f(x)=2x(\arctan 5x)^2+6\tan(\cos 6x)$ .
Solution
$f(x)=2 x(\arctan 5 x)^{2}+6 \tan (\cos 6 x)$ $\frac{d}{d x}\left(\tan ^{-1} 5 x\right)^{2}=2 \tan ^{-1} 5 x\left(\frac{1}{1+(5 x)^{2}}\right)(5)=\frac{10 \tan ^{-1} 5 x}{1+25 x^{2}}$ $\begin{aligned} \frac{d}{d x} 2 x\left(\tan ^{-1} 5 x\right)^{2}&=2 x\left(\frac{10 \tan ^{-1} 5 x}{1+25 x^{2}}\right)+\left(\tan ^{-1} 5 x\right)^{2}(2)\\ &=\frac{20 x \tan ^{-1} 5 x}{1+25 x^{2}}+2\left(\tan ^{-1} 5 x\right)^{2} \end{aligned}$ $\begin{aligned} \frac{d}{d x} 6 \tan (\cos 6 x)&=6 \sec ^{2}(\cos 6 x) \frac{d}{d x} \cos 6 x\\ & =6 \sec ^{2}(\cos 6 x) \times(-\sin 6 x) \times 6 \\ & =-36\left(\sec ^{2}(\cos 6 x)\right) \sin 6 x \end{aligned}$
Therefore, $f^{\prime}(x)=\frac{20 x \tan ^{-1} 5 x}{1+25 x^{2}}+2\left(\tan ^{-1} 5 x\right)^{2}-36\left(\sec ^{2}(\cos 6 x)\right) \sin 6 x$
Solve $y'$ if $y=4x\sinh^{-1}(\frac{x}{6})+\tanh^{-1}(\cos 10x)$ .
Solution
$\begin{aligned}\frac{d}{d x} 4 x \sinh ^{-1}\left(\frac{x}{6}\right)&=4 x\left[\frac{1}{\sqrt{1+\left(\frac{x}{6}\right)^{2}}} \cdot \frac{1}{6}\right]+4 \sinh ^{-1}\left(\frac{x}{6}\right)\\ &=\frac{\frac{2 x}{3}}{\sqrt{1+\frac{x^{2}}{36}}}+4 \sinh ^{-1}\left(\frac{x}{6}\right) \end{aligned}$ $\begin{aligned} \frac{d}{d x} \tanh ^{-1}(\cos 10 x) \quad &=\frac{1}{1-(\cos 10 x)^{2}}\left(\frac{d}{d x} \cos 10 x\right)\\ &=\frac{1}{1-(\cos 10 x)^{2}}(-10 \sin 10 x) \\ &=\frac{-10 \sin 10 x}{1-\cos ^{2} 10 x} \\ &=-\frac{10 \sin ^{2} 10 x}{\sin ^{2} 10 x} \\ &=-\frac{10}{\sin 10 x}\\ &=-10 \csc 10 x \end{aligned}$ $\begin{aligned} \therefore f'(x)=\frac{\frac{2x}{3}}{\sqrt{1+\frac{x^2}{36}}}+4\sinh^{-1}{(\frac{x}{6})}-10\csc{10x} \end{aligned}$
The engineering discipline of piping design studies the efficient transport of fluid. You are required to design a stable pipe that can hold 200 litres of fluid at a time. Assuming that it is a closed ended pipe at both ends, determine the dimensions (radius and height in cm) of the pipe that will minimize the amount of material used to construct the pipe with justification.
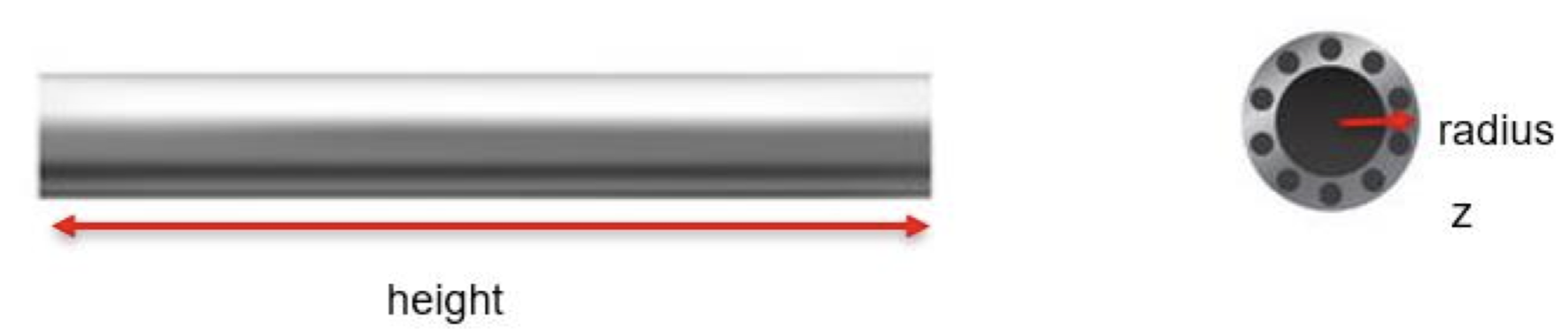Solution
$\begin{align} A&=2\pi r^2+2\pi r h \\ \pi r^2 h&= 200,000 \quad \Rightarrow h=\frac{200,000}{\pi r^2} \end{align}$ $\begin{aligned} A(r) &= 2\pi r^2 + \frac{400,000}{r} \\ A'(r) &= 4\pi r - \frac{400,000}{r^2} \end{aligned}$
Find critical number, $A'(r)=0$ , $\text{Radius, }r = 31.7\text{ cm}$
$A''(r)=4\pi + \frac{800,000}{r^3}$ → +ve → concavity: concave upward → min point.
Hence $h = 63.3 \text{ cm}$ .
You are a manufacturer of metal cones. In order to minimize the material used in the manufacturing process, you need to fabricate the metal cones shown below. You are provided with 3 copper cones with total volume of $15 m^3$ . Each cone consists of the flat base surface and lateral surface. Determine the possible dimensions of the lateral surface for each cone to estimate the amount of copper material needed to construct each cone’s lateral surface. (Given the dimensions of cone are height of the cone, $h$ , the slant length, $s$ , and the radius of the base, $r$ ).
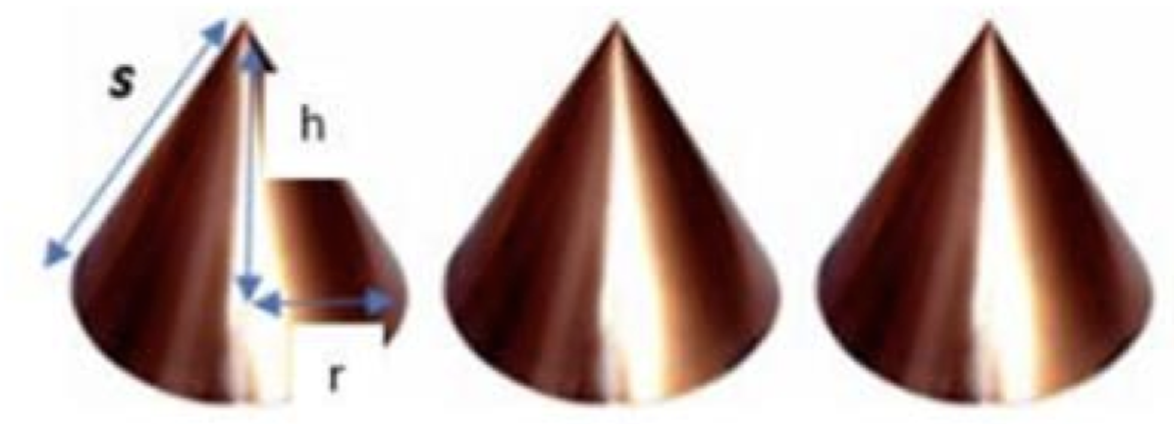Solution
Volume of each cone $=\frac{1}{3} \pi r^{2} h=5, \quad h=\frac{15}{\pi r^{2}}$
Area, $\mathrm{A}(r)$ in terms of $r$ by substituting $h$ .
$\begin{aligned} \mathrm{A}(r)&=\pi r s \quad \text { where } s \text { is the slant length, } \\ \mathrm{A}(r)&=\pi r\left(\sqrt{r^{2}+h^{2}}\right) \\ \mathrm{A}(r)&=\pi r\left(\sqrt{r^{2}+\left(\frac{15}{\pi r^{2}}\right)^{2}}\right) \end{aligned}$
Find $A^{\prime}(r)$
$\begin{aligned} \mathrm{u}(r) &=\pi r \quad \mathrm{u}^{\prime}(r)=\pi \\ \mathrm{v}(r) &=\sqrt{r^{2}+\left(\frac{15}{\pi r^{2}}\right)^{2}} \\ \mathrm{v}^{\prime}(r) &=\frac{1}{2}\left(r^{2}+\frac{15^{2}}{\pi^{2} r^{4}}\right)^{-\frac{1}{2}} \cdot\left(2 r-4\left(\frac{225}{9.87}\right) r^{-5}\right.\\ &=\frac{1}{2}\left(r^{2}+\frac{22.8}{r^{4}}\right)^{-\frac{1}{2}} \cdot\left(2 r-\frac{91.185}{r^{5}}\right) \\ \mathrm{A}^{\prime}(r) &=\frac{1}{2} \pi r\left(r^{2}+\frac{22.8}{r^{4}}\right)^{-\frac{1}{2}} \cdot\left(2 r-\frac{91.185}{r^{5}}\right)+\pi \sqrt{r^{2}+\left(\frac{15}{\pi r^{2}}\right)^{2}} \end{aligned}$
Find critical numbers when $\mathrm{A}^{\prime}(r)=0$ .
$\begin{aligned} \frac{\frac{91.185}{r^{5}} \cdot \frac{1}{2} \pi r-\frac{2 r}{2} \pi r}{\left(r^{2}+\frac{22.8}{r^{4}}\right)^{\frac{1}{2}}}&=+\pi \sqrt{r^{2}+\left(\frac{15}{\pi r^{2}}\right)^{2}} \\ \frac{143.25}{r^{4}}-\pi r^{2}&=\pi\left(r^{2}+\frac{22.8}{r^{4}}\right) \\ \frac{1}{r^{4}}(143.25-71.64)&=2 \pi r^{2} \\ r&=1.5 \mathrm{~m} \end{aligned}$
Hence, dimension :
- Radius, $r=1.5 \mathrm{~m}$ ,
- Height, $\displaystyle h=\frac{15}{\pi r^{2}}=2.1 \mathrm{~m}$
- Slant length, $s=\sqrt{r^{2}+h^{2}}=\sqrt{2.25+4.41}=2.58 \mathrm{~m}$

Your Help needed

This website has received 3000 view in the first two weeks! However, this project repository did not receive any star! Just simply head over to this website's repository to give it a star! Every tutorial takes me around two hours to compile, proof-read and styling this website! Your simple action would encourage me a lot! Thank you! You could Buy me a coffee too!