Skip to main content

Tutorial 14

Tutorial 14: Stokes' Theorem

  1. Suppose F=⟨−y,x,z⟩\mathbf{F}=\langle-y, x, z\rangle and SS is the part of the sphere x2+y2+z2=25x^{2}+y^{2}+z^{2}=25 below the plane z=4\mathrm{z}=4, oriented with the outward-pointing normal (so that the normal at (5,0,0)(5,0,0) is i\mathbf{i} ). Compute the flux integral ∬Scurl F.dS\iint_{S} \text{curl } \mathbf{F} . \mathrm{d} \mathbf{S} using Stokes' theorem.

    Solution

    Again we integrate the line integral over the boundary curve CC rather than the flux integral over the (more complicated) surface SS.

    The boundary curve is the circle x2+y2+42=25x^2+y^2+4^2=25 (or x2+y2=9x^2+y^2=9) in the plane z=4z=4, but a note of caution is in order.

    The natural parameterization (or the one we usually think of) is r(t)=⟨3cos⁡(t),3sin⁡(t),4⟩\mathbf{r}(t) = \langle 3 \cos(t), 3 \sin(t), 4\rangle actually parameterizes −C-C (that is, CC with the opposite orientation)!

    Why is that? Imagine a person walking this boundary with their head in the normal (outward) direction. The remaining part of the sphere is on their right if they’re walking counter-clockwise. It should be on their left, so they should be walking clockwise.

    We’ll calculate ∮−CF⋅dr\oint_{-C}\mathbf{F}\cdot d\mathbf{r} anyway, since we like the parameterisation. In terms of this parametrisation,

    F(r(t))=⟨−3sin⁡(t),3cos⁡(t),4⟩dr(t)=⟨−3sin⁡(t),3cos⁡(t),0⟩dtF(r(t))⋅dr(t)=9dt\begin{equation*} \begin{aligned} \mathbf{F}(\mathbf{r}(t))&=\langle-3\sin(t),3\cos(t),4\rangle\\ d\mathbf{r}(t)&=\langle-3\sin(t),3\cos(t),0\rangle dt\\ \mathbf{F}(\mathbf{r}(t))\cdot d\mathbf{r}(t)&=9dt \end{aligned} \end{equation*}

    Thus,

    ∫−CF⋅dr=∫02π9dt=18π\int_{-C}\mathbf{F}\cdot d\mathbf{r}=\int_0^{2\pi}9dt=18\pi

    and so, ∫CF⋅dr=−∫−CF⋅dr=−18π\displaystyle\int_C \mathbf{F}\cdot d\mathbf{r}=-\int_{-C}\mathbf{F}\cdot d\mathbf{r}=-18\pi.

  2. Use Stokes' theorem to evaluate ∬Scur∣F.dS\iint_{S} c u r \mid \mathbf{F} . \mathrm{d} \mathbf{S} where F=z2i−3xyj+x3y3kF=z^{2} \mathbf{i}-3 x y \mathbf{j}+x^{3} y^{3} \mathbf{k} and S\mathrm{S} is the part of z=5−x2−y2z=5-x^{2}-y^{2} above the z=1z=1. Assume that SS is oriented upwards.

    Solution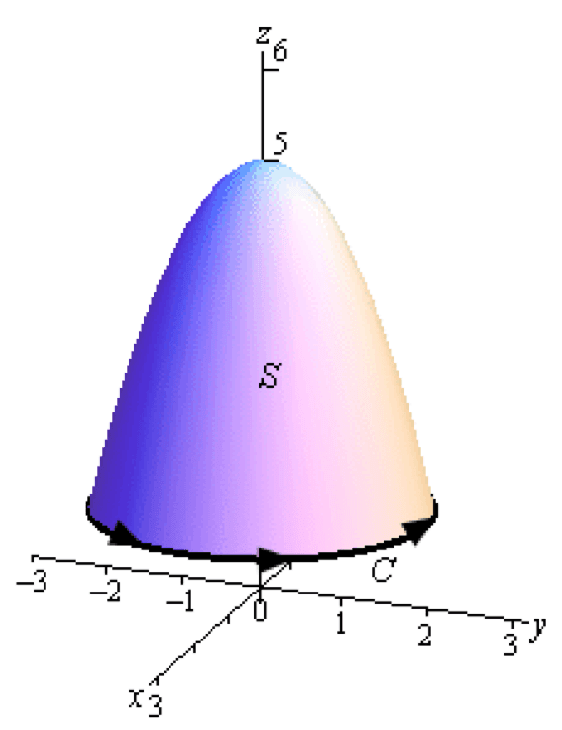
    Figure for Question 2

    In this case the boundary curve CC will be where the surface intersects the plane z=1z=1 and so will be the curve

    1=5−x2−y2x2+y2=4at z=1\begin{equation*} \begin{aligned} 1 & = 5 - {x^2} - {y^2}\\ {x^2} + {y^2} & = 4\quad\text{at }z=1 \end{aligned} \end{equation*}

    So, the boundary curve will be the circle of radius 2 that is in the plane z=1z=1. The parameterization of this curve is,

    r⃗(t)=2cos⁡t i⃗+2sin⁡t j⃗+k⃗,    0≤t≤2π\vec r\left( t \right) = 2\cos t\,\vec i + 2\sin t\,\vec j + \vec k,\,\,\,\,0 \le t \le 2\pi

    The first two components give the circle and the third component makes sure that it is in the plane z=1z=1.

    Using Stokes’ Theorem we can write the surface integral as the following line integral.

    ∬ScurlF⃗ ⋅ dS⃗=∫CF⃗ ⋅ d r⃗=∫ 0 2πF⃗(r⃗(t)) ⋅ r⃗′(t) dt\iint\limits_{S}{{{\mathop{\rm curl}\nolimits} \vec F\,\centerdot \,d\vec S}} = \int\limits_{C}{{\vec F\,\centerdot \,d\,\vec r}} = \int_{{\,0}}^{{\,2\pi }}{{\vec F\left( {\vec r\left( t \right)} \right)\,\centerdot \,\vec r'\left( t \right)\,dt}}

    So, it looks like we need a couple of quantities before we do this integral. Let’s first get the vector field evaluated on the curve. Remember that this is simply plugging the components of the parameterization into the vector field.

    F⃗(r⃗(t))=(1)2 i⃗−3(2cos⁡t)(2sin⁡t) j⃗+(2cos⁡t)3(2sin⁡t)3 k⃗=i⃗−12cos⁡tsin⁡t j⃗+64cos⁡3tsin⁡3t k⃗\begin{equation*} \begin{aligned} \vec F\left( {\vec r\left( t \right)} \right) & = {\left( 1 \right)^2}\,\vec i - 3\left( {2\cos t} \right)\left( {2\sin t} \right)\,\vec j + {\left( {2\cos t} \right)^3}{\left( {2\sin t} \right)^3}\,\vec k\\ & = \vec i - 12\cos t\sin t\,\vec j + 64{\cos ^3}t{\sin ^3}t\,\vec k \end{aligned} \end{equation*}

    Next, we need the derivative of the parameterization and the dot product of this and the vector field.

    r⃗′(t)=−2sin⁡t i⃗+2cos⁡t j⃗F⃗(r⃗(t)) ⋅ r⃗′(t) =−2sin⁡t−24sin⁡tcos⁡2t\begin{equation*} \begin{aligned} \vec r'\left( t \right) & = - 2\sin t\,\vec i + 2\cos t\,\vec j\\ \vec F\left( {\vec r\left( t \right)} \right)\,\centerdot \,\vec r'\left( t \right)\, & = - 2\sin t - 24\sin t{\cos ^2}t \end{aligned} \end{equation*}

    We can now do the integral.

    ∬ScurlF⃗ ⋅ dS⃗=∫ 0 2π−2sin⁡t−24sin⁡tcos⁡2t dt=(2cos⁡t+8cos⁡3t)∣02π=0\begin{equation*} \begin{aligned} \iint\limits_{S}{{{\mathop{\rm curl}\nolimits} \vec F\,\centerdot \,d\vec S}} & = \int_{{\,0}}^{{\,2\pi }}{{ - 2\sin t - 24\sin t{{\cos }^2}t\,dt}}\\ & = \left. {\left( {2\cos t + 8{{\cos }^3}t} \right)} \right|_0^{2\pi }\\ & = 0 \end{aligned} \end{equation*}

  3. Use Stokes' theorem to evaluate ∫cF.dr\int_{c} \mathbf{F} . d \mathbf{r} where F=z2i+y2j+xk\mathbf{F}=z^{2} \mathbf{i}+y^{2} \mathbf{j}+x \mathbf{k} and CC is the triangle with vertices (1,0,0),(0,1,0)(1,0,0),(0,1,0) and (0,0,1)(0,0,1) with counter clockwise rotation.

    Solution

    We are going to need the curl of the vector field eventually so let’s get that out of the way first.

    curlF⃗=∣i⃗j⃗k⃗∂∂x∂∂y∂∂zz2y2x∣=2z j⃗−j⃗=(2z−1)j⃗{\mathop{\rm curl}\nolimits} \vec F = \left| {\begin{matrix}{\vec i}&{\vec j}&{\vec k}\\{\frac{\partial }{{\partial x}}}&{\frac{\partial }{{\partial y}}}&{\frac{\partial }{{\partial z}}}\\{{z^2}}&{{y^2}}&x\end{matrix}} \right| = 2z\,\vec j - \vec j = \left( {2z - 1} \right)\vec j

    Now, all we have is the boundary curve for the surface that we’ll need to use in the surface integral. However, as noted above all we need is any surface that has this as its boundary curve. So, let’s use the following plane with upwards orientation for the surface.

    Figure for Question 3

    Since the plane is oriented upwards this induces the positive direction on CC as shown. The equation of this plane is,

    x+y+z=1   ⇒   z=g(x,y)=1−x−yx + y + z = 1\hspace{0.25in}\,\,\, \Rightarrow \hspace{0.25in}\,\,\,z = g\left( {x,y} \right) = 1 - x - y

    Now, let’s use Stokes’ Theorem and get the surface integral set up.

    ∫CF⃗ ⋅ d r⃗=∬ScurlF⃗ ⋅ dS⃗=∬S(2z−1)j⃗⋅dS⃗=∬D(2z−1)j⃗⋅∇f∥∇f∥  ∥∇f∥ dA\begin{equation*} \begin{aligned} \int\limits_{C}{{\vec F\,\cdot \,d\,\vec r}} & = \iint\limits_{S}{{{\mathop{\rm curl}\nolimits} \vec F\,\centerdot \,d\vec S}}\\ & = \iint\limits_{S}{{\left( {2z - 1} \right)\vec j\cdot d\vec S}}\\ & = \iint\limits_{D}{{\left( {2z - 1} \right)\vec j\cdot \frac{{\nabla f}}{{\left\| {\nabla f} \right\|}}\,\,\left\| {\nabla f} \right\|\,dA}}\end{aligned} \end{equation*}

    Okay, we now need to find a couple of quantities. First let’s get the gradient. Recall that this comes from the function of the surface.

    f(x,y,z)=z−g(x,y)=z−1+x+y∇f=i⃗+j⃗+k⃗\begin{equation*} \begin{aligned} f\left( {x,y,z} \right) &= z - g\left( {x,y} \right) = z - 1 + x + y\\ & \nabla f = \vec i + \vec j + \vec k \end{aligned} \end{equation*}

    Note as well that this also points upwards and so we have the correct direction.

    Now, DD is the region in the xyxy-plane shown below,

    Figure for Question 3

    We get the equation of the line by plugging in z=0z=0 into the equation of the plane. So based on this the ranges that define DD are,

    0≤x≤10≤y≤−x+10 \le x \le 1\hspace{0.25in}0 \le y \le - x + 1

    The integral is then,

    ∫CF⃗ ⋅ d r⃗=∬D(2z−1)j⃗⋅(i⃗+j⃗+k⃗) dA=∫ 0 1∫ 0−x+12(1−x−y)−1 dy dx\begin{equation*} \begin{aligned} \int\limits_{C}{{\vec F\,\cdot \,d\,\vec r}} & = \iint\limits_{D}{{\left( {2z - 1} \right)\vec j\cdot \left( {\vec i + \vec j + \vec k} \right)\,dA}}\\ & = \int_{{\,0}}^{{\,1}}{{\int_{{\,0}}^{{ - x + 1}}{{2\left( {1 - x - y} \right) - 1\,dy}}\,dx}} \end{aligned} \end{equation*}

    Don’t forget to plug in for zz since we are doing the surface integral on the plane. Finishing this out gives,

    ∫CF⃗ ⋅ d r⃗=∫ 0 1∫ 0−x+11−2x−2y dy dx=∫ 0 1(y−2xy−y2)∣0−x+1 dx=∫ 0 1x2−x dx=(13x3−12x2)∣01=−16\begin{equation*} \begin{aligned} \int\limits_{C}{{\vec F\,\cdot \,d\,\vec r}} & = \int_{{\,0}}^{{\,1}}{{\int_{{\,0}}^{{ - x + 1}}{{1 - 2x - 2y\,dy}}\,dx}}\\ & = \int_{{\,0}}^{{\,1}}{{\left. {\left( {y - 2xy - {y^2}} \right)} \right|_0^{ - x + 1}\,dx}}\\ & = \int_{{\,0}}^{{\,1}}{{{x^2} - x\,dx}}\\ & = \left. {\left( {\frac{1}{3}{x^3} - \frac{1}{2}{x^2}} \right)} \right|_0^1\\ & = - \frac{1}{6} \end{aligned} \end{equation*}

  4. Verify Stokes' Theorem for the field F=⟨x2,2x,z2⟩\boldsymbol{F}=\left\langle x^{2}, 2 x, z^{2}\right\rangle on the ellipse S={(x,y,z):4x2+y2≤4,z=0}S=\left\{(x, y, z): 4 x^{2}+y^{2} \leq 4, z=0\right\}

    Solution

    We compute both sides in ∮CF⋅dr=∬S(∇×F)⋅ndσ\oint_C\mathbf{F}\cdot d\mathbf{r}=\iint_S(\nabla\times\mathbf{F})\cdot\mathbf{n}d\sigma

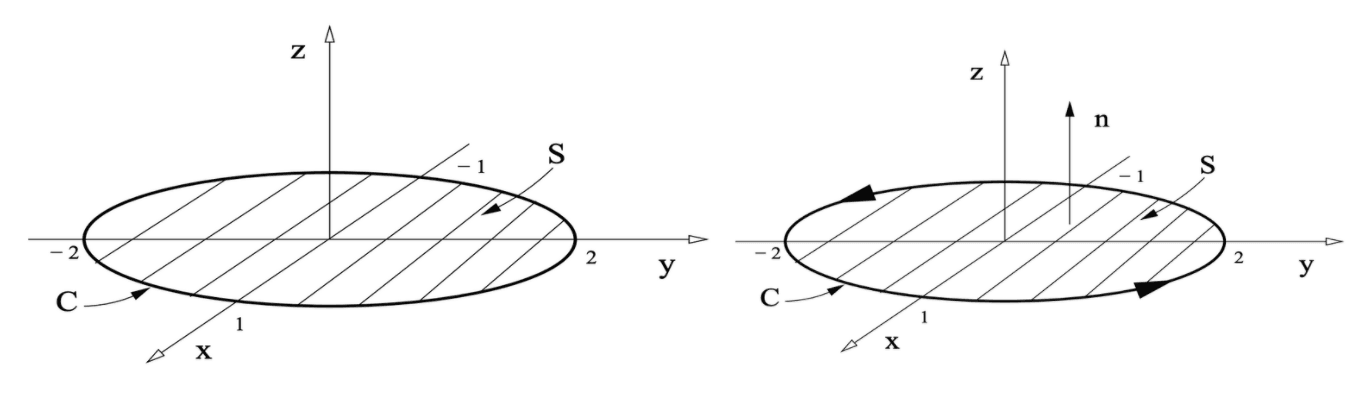
    Figure for Question 4

    We start computing the circulation integral on the ellipse x2+y222=1x^2+\frac{y^2}{2^2}=1. We need to choose a counterclockwise parametrization, hence the normal to SS points upwards.

    We choose, for t∈[0,2π]t \in [0, 2\pi],

    r(t)=⟨cos⁡(t),2sin⁡(t),0⟩r(t)=\langle \cos{(t)},2\sin(t),0\rangle

    Therefore, the right-hand rule normal n\mathbf{n} to SS is n=⟨0,0,1⟩\mathbf{n} = \langle 0, 0, 1\rangle

    The circulation integral is:

    ∮CF⋅dr=∫02πF(t)⋅r′(t)dt=∫02π⟨cos⁡2(t),2cos⁡(t),0⟩⋅⟨−sin⁡(t),2cos⁡(t),0⟩dt=∫02π[−cos⁡2(t)sin⁡(t)+4cos⁡2(t)]dt\begin{equation*} \begin{aligned} \oint_C\mathbf{F}\cdot d\mathbf{r}&=\int_0^{2\pi} \mathbf{F}(t)\cdot \mathbf{r}'(t)dt\\ &=\int_0^{2\pi}\langle\cos^2(t),2\cos(t),0\rangle\cdot\langle-\sin(t),2\cos(t),0\rangle dt\\ &=\int_0^{2\pi}[-\cos^2(t)\sin(t)+4\cos^2(t)]dt \end{aligned} \end{equation*}

    The substitution on the first term u=cos⁡(t)u = \cos(t) and du=−sin⁡(t)dtdu = -\sin(t) dt, implies ∫02π−cos⁡2(t)sin⁡(t)dt=∫11u2du=0\int_0^{2\pi} -\cos^2(t)\sin(t)dt=\int_1^1 u^2du=0.

    ∮CF⋅dr=∫02π4cos⁡2(t)dt=∫02π2[1+cos⁡(2t)]dt\oint_C\mathbf{F}\cdot d\mathbf{r}=\int_0^{2\pi}4\cos^2(t)dt=\int_0^{2\pi}2[1+\cos(2t)]dt

    Since ∫02πcos⁡(2t)dt=0\displaystyle\int_0^{2\pi}\cos(2t)dt=0, we conclude that ∮CF⋅dr=4π\displaystyle\oint_C\mathbf{F}\cdot d\mathbf{r}=4\pi

    We now compute the right-hand side in Stokes’ Theorem.

    Figure for Question 4
    I=∬S(∇×F)⋅ndσI=\iint_S(\nabla\times\mathbf{F})\cdot\mathbf{n}d\sigma
    ∇×F=∣ijk∂x∂y∂zx22xz2∣⇒∇×F=⟨0,0,2⟩\nabla\times\mathbf{F}=\left|\begin{matrix}\mathbf{i}&\mathbf{j}&\mathbf{k}\\\partial_x&\partial_y&\partial_z\\x^2&2x&z^2\end{matrix}\right| \quad \Rightarrow \quad \nabla\times\mathbf{F}=\langle 0,0,2 \rangle

    SS is the flat surface {x2+y222≤1,z=0}\{x^2+\frac{y^2}{2^2}\le1,\quad z=0\}, so dσ=dxdyd\sigma=dxdy

    Then, ∬S(∇×F)⋅ndσ=∫−11∫−21−x221−x2⟨0,0,2⟩⋅⟨0,0,1⟩dydx\displaystyle\iint_S(\nabla\times\mathbf{F})\cdot\mathbf{n}d\sigma=\int_{-1}^1\int_{-2\sqrt{1-x^2}}^{2\sqrt{1-x^2}}\langle0,0,2\rangle\cdot\langle0,0,1\rangle dydx

    The right-hand side above is twice the area of the ellipse, Since that an ellipse x2a2+y2b2=1\displaystyle\frac{x^2}{a^2}+\frac{y^2}{b^2}=1 has area πab\pi ab, we obtain

    ∬S(∇×F)⋅ndσ=4π\iint_S(\nabla\times\mathbf{F})\cdot\mathbf{n}d\sigma=4\pi

  5. Verify Stokes' Theorem for F=⟨y2,−x,5z⟩\boldsymbol{F}=\left\langle y^{2},-x, 5 z\right\rangle and SS is the paraboloid z=x2+y2z=x^{2}+y^{2} with the circle x2+y2=1x^{2}+y^{2}=1 as its boundary.

    Solution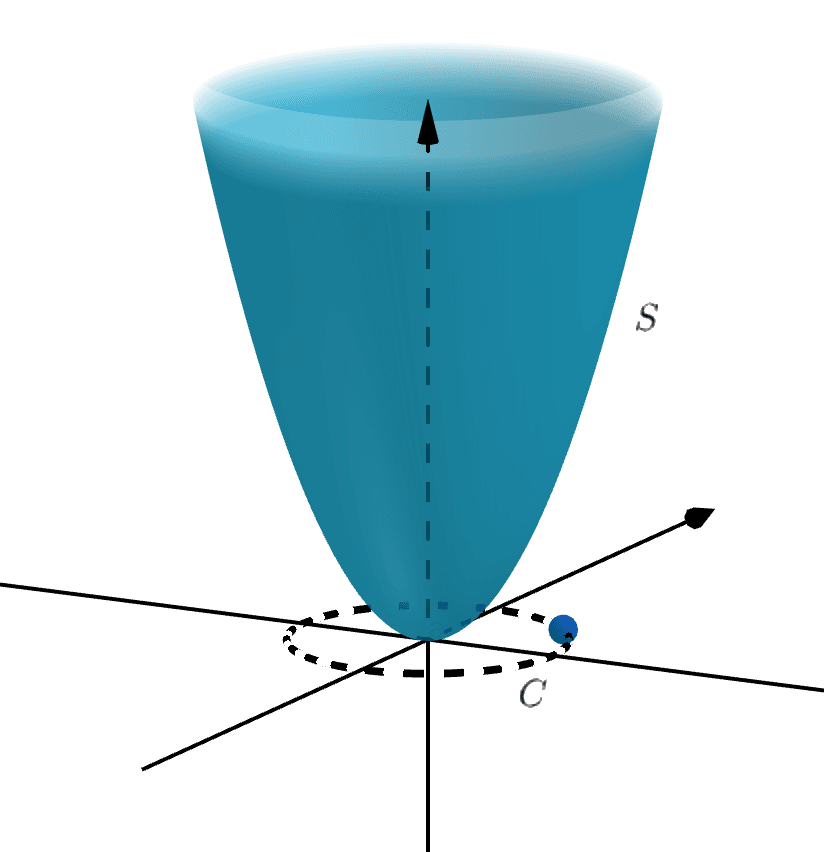
    Figure for Question 5

    Surface integral,

    ∇×F⃗=curl F⃗=∣i^j^k^∂∂x∂∂y∂∂zy2−x5z∣=i^(0−0)−j^(0−0)+k^(−1−2y)=(−1−2y)k^\begin{equation*} \begin{aligned} \nabla\times\vec{F}=\text{curl }\vec{F}&=\left|\begin{matrix}\hat{i}&\hat{j}&\hat{k}\\ \frac{\partial}{\partial x}&\frac{\partial}{\partial y}&\frac{\partial}{\partial z}\\y^2&-x&5z\end{matrix}\right|\\ &=\hat{i}(0-0)-\hat{j}(0-0)+\hat{k}(-1-2y)\\ &=(-1-2y)\hat{k} \end{aligned} \end{equation*}
    n⃗=⟨fx,fy,1⟩or⟨−fx,−fy,1⟩=⟨−2x,−2y,1⟩\begin{equation*} \begin{aligned} \vec{n}&=\langle f_x,f_y,1\rangle \quad \text{or}\quad \langle-f_x,-f_y,1\rangle\\ &=\langle -2x,-2y,1\rangle \end{aligned} \end{equation*}
    (∇×F⃗)⋅n⃗=0(−2x)+0(−2y)+(−1−2y)(1)=−1−2y(\nabla\times\vec{F})\cdot\vec{n}=0(-2x)+0(-2y)+(-1-2y)(1)=-1-2y
    ∬R(∇×F⃗)⋅n⃗dxdy=∬R(−1−2y)dxdy\iint_R(\nabla\times\vec{F})\cdot\vec{n}dxdy=\iint_R(-1-2y)dxdy

    RR is region in side CC, a unit circle.

    Switching to polar coordinates:

    x=rcos⁡θ,y=rsin⁡θ,r∈[0,1],θ∈[0,2π]x=r\cos\theta,\quad y=r\sin\theta,\quad r\in[0,1],\quad\theta\in[0,2\pi]

    Hence,

    =∫02π∫01(−1−2rsin⁡θ)rdrdθ=∫02π(−r22−2sin⁡θ(r33))∣01dθ=∫02π(−12−23sin⁡θ)dθ=(−12θ+23cos⁡θ)∣02π=−12(2π−0)+23(cos⁡(2π)−cos⁡0)=−π\begin{equation*} \begin{aligned} &=\int_0^{2\pi}\int_0^1(-1-2r\sin\theta)rdrd\theta\\ &=\int_0^{2\pi}\left.\left(-\frac{r^2}{2}-2\sin\theta\left(\frac{r^3}{3}\right)\right)\right|_0^1d\theta\\ &=\int_0^{2\pi}\left(-\frac{1}{2}-\frac{2}{3}\sin\theta\right)d\theta\\ &=\left.\left(-\frac{1}{2}\theta+\frac{2}{3}\cos\theta\right)\right|_0^{2\pi}\\ &=-\frac{1}{2}(2\pi-0)+\frac{2}{3}(\cos(2\pi)-\cos0)\\ &=-\pi \end{aligned} \end{equation*}

    Line integral,

    ∮CF⃗⋅T⃗ds=∫CMdx+Ndy+Pdz=∫Cy2dx−xdy+5zdz\begin{equation*} \begin{aligned} \oint_C\vec{F}\cdot\vec{T}ds&=\int_CMdx+Ndy+Pdz\\ &=\int_Cy^2dx-xdy+5zdz \end{aligned} \end{equation*}

    CC: unit circle →\rightarrow Switch to polar coordinates,

    x=cos⁡θ,y=sin⁡θ,z=1dx=−sin⁡θdθ,dy=cos⁡θdθ,dz=0\begin{equation*} \begin{aligned} x&=\cos\theta,\qquad \quad &y&=\sin\theta,\qquad &z&=1\\ dx&=-\sin\theta d\theta,\quad &dy&=\cos\theta d\theta,\quad &dz&=0 \end{aligned} \end{equation*}

    Hence,

    =∫02π(sin⁡2θ)(−sin⁡θ)dθ−cos⁡2θdθ+0dθ=∫02π(−sin⁡3θ−cos⁡2θ)dθ=∫02π(−sin⁡3θ)dθ−∫02πcos⁡2θdθ=−12∫02π(1+cos⁡(2θ))dθ=−12(θ+sin⁡(2θ)2)∣02π=−12(2π−0)=−π\begin{equation*} \begin{aligned} &=\int_0^{2\pi}(\sin^2\theta)(-\sin\theta)d\theta-\cos^2\theta d\theta+0d\theta\\ &=\int_0^{2\pi}(-\sin^3\theta-\cos^2\theta)d\theta\\ &=\int_0^{2\pi}(-\sin^3\theta)d\theta-\int_0^{2\pi}\cos^2\theta d\theta\\ &=-\frac{1}{2}\int_0^{2\pi}(1+\cos(2\theta))d\theta\\ &=-\frac{1}{2}\left.\left(\theta+\frac{\sin(2\theta)}{2}\right)\right|_0^{2\pi}\\ &=-\frac{1}{2}(2\pi-0)\\ &=-\pi \end{aligned} \end{equation*}

  6. Use Stokes' Theorem to calculate ∬(∇×F)⋅n^dS\iint(\nabla \times \boldsymbol{F}) \cdot \hat{n} d S for F=⟨xz2,x3,cos⁡(xz)⟩\boldsymbol{F}=\left\langle x z^{2}, x^{3}, \cos (x z)\right\rangle where SS is the part of the ellipsoid xx2+y2+3z2=1x x^{2}+y^{2}+3 z^{2}=1 below the xyx y-plane and n^\hat{n} is the lower normal.

    Solution
    ∬S(∇×F⃗)⋅n^dS=∮CF⃗⋅T⃗dS=∫CMdx+Ndy+Pdz=∫Cxz2dx+x3dy+cos⁡(xz)dz\begin{equation*} \begin{aligned} \iint_S(\nabla\times\vec{F})\cdot\hat{n}dS&=\oint_C\vec{F}\cdot\vec{T}dS\\ &=\int_CMdx+Ndy+Pdz\\ &=\int_Cxz^2dx+x^3dy+\cos(xz)dz \end{aligned} \end{equation*}

    Using polar coordinates,

    z=0,x=cos⁡θ,y=sin⁡θdz=0,dx=−sin⁡θdθ,dy=cos⁡θdθ\begin{equation*} \begin{aligned} z&=0,\quad &x&=\cos\theta,\qquad \quad &y&=\sin\theta\\ dz&=0,\quad &dx&=-\sin\theta d\theta, \quad &dy&=\cos\theta d\theta \end{aligned} \end{equation*}

    Hence,

    =∫02πcos⁡θ(0)(−sin⁡θdθ)+cos⁡3θ(cos⁡θ)dθ+0=∫02πcos⁡4θdθ=3π4\begin{equation*} \begin{aligned} &=\int_0^{2\pi}\cos\theta(0)(-\sin\theta d\theta)+\cos^3\theta(\cos\theta)d\theta+0\\ &=\int_0^{2\pi}\cos^4\theta d\theta=\frac{3\pi}{4} \end{aligned} \end{equation*}

  7. Use Stoke's Theorem to evaluate the line integral ∫F⋅drc\int \boldsymbol{F} \cdot d \boldsymbol{r} c where F\boldsymbol{F} is the vector F=\boldsymbol{F}= (4ex2−y)i+(16sin⁡(y2)+3x)j+(4y−2x−ez)k\left(4 e^{x^{2}}-y\right) \boldsymbol{i}+\left(16 \sin \left(y^{2}\right)+3 x\right) \boldsymbol{j}+\left(4 y-2 x-e^{z}\right) \boldsymbol{k} and CC is the curve of intersection of the cylinder x2+y2=16x^{2}+y^{2}=16 and the plane z=2x+4yz=2 x+4 y and CC is oriented in a counterclockwise direction when viewed from above.

    Solution

    The curl\text{curl} of F\mathbf{F} is computed as,

    curl F=∣ijk∂∂x∂∂y∂∂z4ex2−1y16sin⁡(y2)+3x4y−2x−ez∣=−4i−2j+4k\begin{equation*} \begin{aligned} \text{curl }\mathbf{F}&=\left|\begin{matrix}\mathbf{i}&\mathbf{j}&\mathbf{k}\\\frac{\partial}{\partial x}&\frac{\partial}{\partial y}&\frac{\partial}{\partial z}\\ 4e^{x^2}-1y&16\sin(y^2)+3x&4y-2x-e^z\end{matrix}\right|\\&=-4\mathbf{i}-2\mathbf{j}+4\mathbf{k} \end{aligned} \end{equation*}

    Now, writing the plane z=2x+4yz=2x+4y as the level surface G(x,y,z)=−2x−4y+z=0G(x,y,z)=-2x-4y+z=0,

    NdS=∇GdA=⟨−2,−4,1⟩dA\mathbf{N}dS=\nabla GdA=\langle-2,-4,1\rangle dA

    Applying Stokes’ theorem,

    ∫CF⋅dr=∫S∫⟨−4,−2,4⟩⋅⟨−2,−4,1⟩dA=∫R∫[(−4)(−2)+(−2)(−4)+(4)(1)]dA=∫R∫20dA=(20)(Area of R)=(20)(16π)=320π\begin{equation*} \begin{aligned} \int_C\mathbf{F}\cdot d\mathbf{r}&=\int_S\int\langle-4,-2,4\rangle\cdot\langle-2,-4,1\rangle dA\\ &=\int_R\int[(-4)(-2)+(-2)(-4)+(4)(1)]dA\\&=\int_R\int20dA\\ &=(20)(\text{Area of }R)\\&=(20)(16\pi)=320\pi \end{aligned} \end{equation*}

  8. Evaluate the line integral of F(x,y,z)=⟨xy,2z,3y⟩\boldsymbol{F}(x, y, z)=\langle x y, 2 z, 3 y\rangle over the curve CC that is the intersection of the cylinder x2+y2=9x^{2}+y^{2}=9 with the plane x+z=5x+z=5.

    Solution

    To describe the surface SS enclosed by CC, we use the parameterisation

    x=ucos⁡v,y=usin⁡v,z=5−ucos⁡v,0≤u≤3,0≤v≤2πx=u\cos v,\quad y=u\sin v,\quad z=5-u\cos v,\quad0\le u\le3,\quad 0\le v\le2\pi

    Using ru=⟨cos⁡v,sin⁡v,−cos⁡v⟩\mathbf{r}_u=\langle\cos v,\sin v,-\cos v\rangle and rv=⟨−usin⁡v,ucos⁡v,usin⁡v⟩\mathbf{r}_v=\langle-u\sin v,u\cos v,u\sin v\rangle, we obtain,

    ru×rv=⟨u,0,u⟩\mathbf{r}_u\times\mathbf{r}_v=\langle u,0,u\rangle

    Compute the curl,

    curl F=⟨∂∂x,∂∂y,∂∂z⟩×⟨xy,2z,3y⟩=⟨1,0,x⟩\text{curl }\mathbf{F}=\left\langle\frac{\partial}{\partial x},\frac{\partial}{\partial y},\frac{\partial}{\partial z}\right\rangle\times\langle xy,2z,3y\rangle=\langle1,0,x\rangle

    Let DD be the domain of the parameter,

    D={(u,v)∣0≤u≤3,0≤v≤2π}D=\{(u,v)|0\le u\le 3,\quad 0\le v\le2\pi\}

    Using Green’s Theorem,

    ∫CF⋅dr=∬Scurl F⋅dS=∬Dcurl F(r(u,v))⋅(ru×rv)dA=∫03∫02π⟨1,0,ucos⁡v⟩⋅⟨u,0,u⟩dA=∫03∫02πu+u2cos⁡vdvdu=∫03(uv+u2sin⁡v)∣02πdvdu=2π∫03udvdu=2πu22∣03=9π\begin{equation*} \begin{aligned} \int_C\mathbf{F}\cdot d\mathbf{r}&=\iint_S\text{curl }\mathbf{F}\cdot d\mathbf{S}\\ &=\iint_D\text{curl }\mathbf{F}(\mathbf{r}(u,v))\cdot(\mathbf{r}_u\times\mathbf{r}_v)dA\\ &=\int_0^3\int_0^{2\pi}\langle1,0,u\cos v\rangle\cdot\langle u,0,u\rangle dA\\ &=\int_0^3\int_0^{2\pi}u+u^2\cos vdvdu\\&=\int_0^3(uv+u^2\sin v)|_0^{2\pi}dvdu\\ &=2\pi\int_0^3udvdu\\ &=2\pi\left.\frac{u^2}{2}\right|_0^3\\ &=9\pi \end{aligned} \end{equation*}

  9. Evaluate ∬(∇×F)⋅ndS\iint(\nabla \times \boldsymbol{F}) \cdot \boldsymbol{n} d S where F(x,y,z)=⟨yz,xz,xy⟩\boldsymbol{F}(x, y, z)=\langle y z, x z, x y\rangle and SS is the part of the sphere x2+y2+z2=4x^{2}+y^{2}+z^{2}=4 that lies inside the cylinder x2+y2=1x^{2}+y^{2}=1 and above the xyx y-plane.

    Solution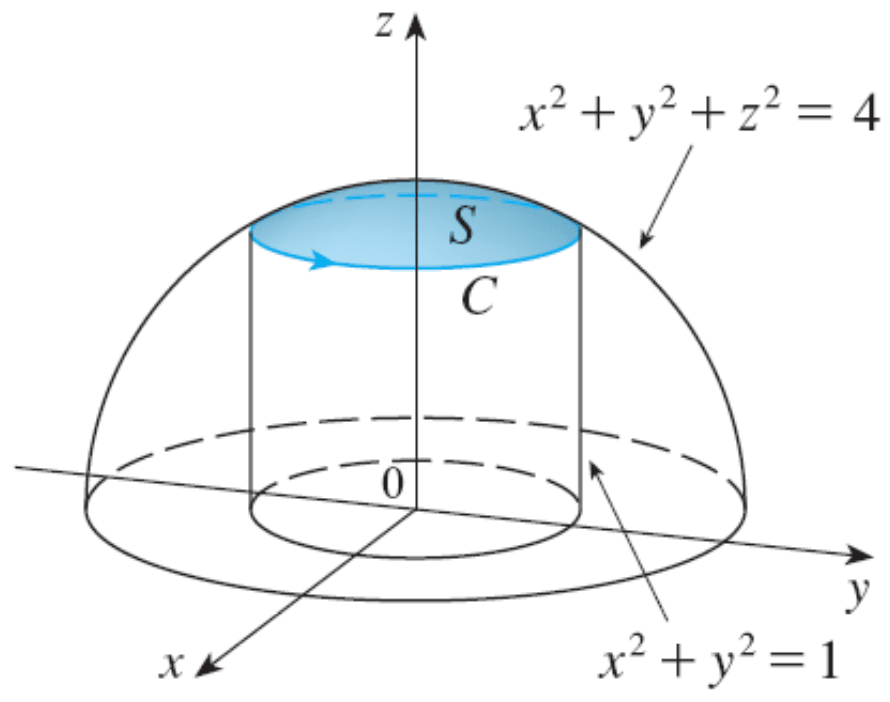
    Figure for Question 9

    Surface SS is bounded by a circle formed by the intersection of the sphere of radius 2 and the cylinder of radius 1.

    We can describe ∂S\partial S using the vector-valued function,

    r(t)=⟨cos⁡t,sin⁡t,3⟩,0≤t≤2π\mathbf{r}(t)=\langle\cos t,\sin t, \sqrt{3}\rangle,\quad 0\le t \le 2\pi

    Using Stokes’ Theorem,

    ∬S(∇×F)⋅ndS=∮∂SF⋅dr=∫02πF(cos⁡t,sin⁡t,3)⋅⟨−sin⁡t,cos⁡t,0⟩dt=3∫02π(cos⁡2t−sin⁡2t)dt=3∫02πcos⁡2tdt=0\begin{equation*} \begin{aligned} \iint_S(\nabla\times\mathbf{F})\cdot\mathbf{n}dS&=\oint_{\partial S}\mathbf{F}\cdot d\mathbf{r}\\ &=\int_0^{2\pi}\mathbf{F}(\cos t,\sin t,\sqrt{3})\cdot\langle-\sin t,\cos t,0\rangle dt\\ &=\sqrt{3}\int_0^{2\pi}(\cos^2 t-\sin^2 t)dt\\ &=\sqrt{3}\int_0^{2\pi}\cos 2tdt\\ &=0\end{aligned} \end{equation*}