Tutorial 11
Tutorial 11: Multiple Integrals in Polar Coordinate & Its Engineering Application
In the following exercises, change the cartesian integral into an equivalent polar coordinate integral. Then solve the integral in polar coordinate:
a.
SolutionLet , . Therefore, .
b.
Solutionc.
SolutionUse the substitution , so .
When , and when . Thus,
Therefore,
d.
SolutionThe limits of integration are given by and . The upper limit for can be rewritten as , which simplifies to . This inequality describes the area inside and on a circle centered at the origin with a radius of .
Given that and , the region of integration is the portion of this circle in the first quadrant.
The circular nature of the domain and the form of the integrand () make it ideal to convert to polar coordinates. The conversion formulas are:
The limits for the region in polar coordinates become:
- Radius:
- Angle:
The integrand becomes .
Thus, the integral in polar coordinates is:
Next, we evaluate the integral with respect to using integration by parts, where .
Let and . Then and .
Finally, we substitute the result from the inner integral into the outer integral and evaluate with respect to :
Evaluate the using polar coordinates
SolutionThe region is a unit circle, so we can describe it as .
Using the conversion , and , we have
Find the volume below , above -plane and between cylinder and
Solution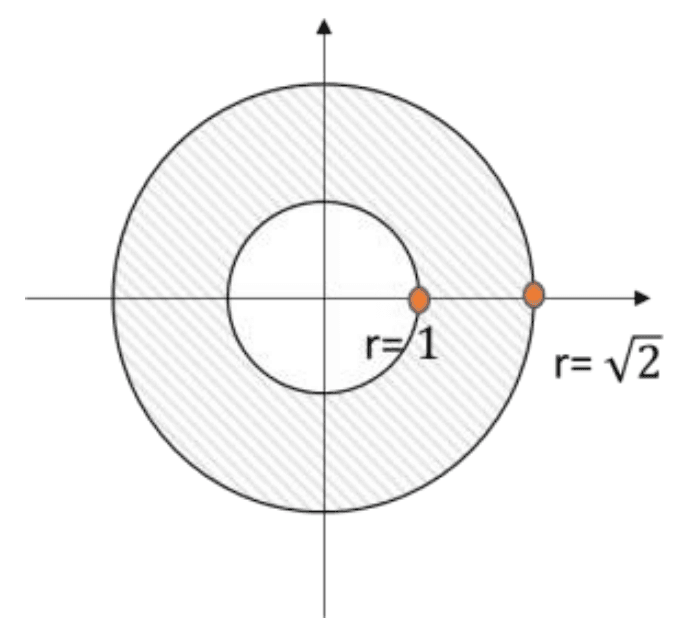
Find the volume between the sphere and the cone
SolutionWe seek the volume inside the sphere and above the cone .
In spherical coordinates , , the volume element is
.
The cone becomes .
Thus, the region is .
Volume is equal to area only if the height () is equal to 1 . Find the area of where is the region bound by .
SolutionRegion bound by
How to sketch the region ?
Volume = area when . Hence,
, a solid is bound by in the first octant
SolutionUse cylindrical coordinates to find the volume of a curved wedge cut out from a cylinder by the planes and .
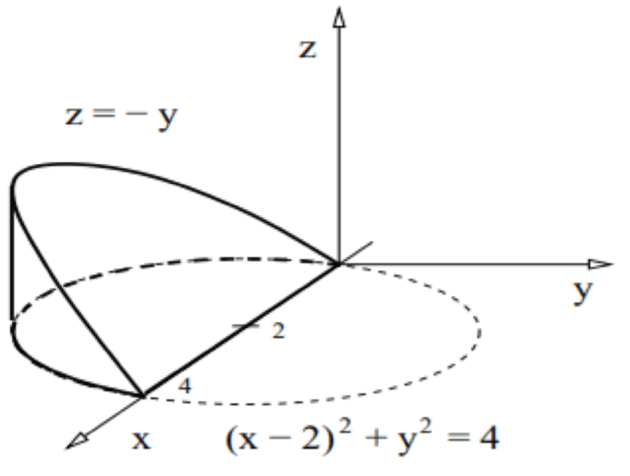 Solution
SolutionFirst, sketch the integration region.
is a circle, since
Since , the integration region is on the part of the plane.
Introduce the substitution: , ;
Consider the region inside the right circular cylinder with equation , bounded below by the -plane and bounded above by the sphere with radius 4 centered at the origin. Set up a triple integral over this region with a function in cylindrical coordinates.
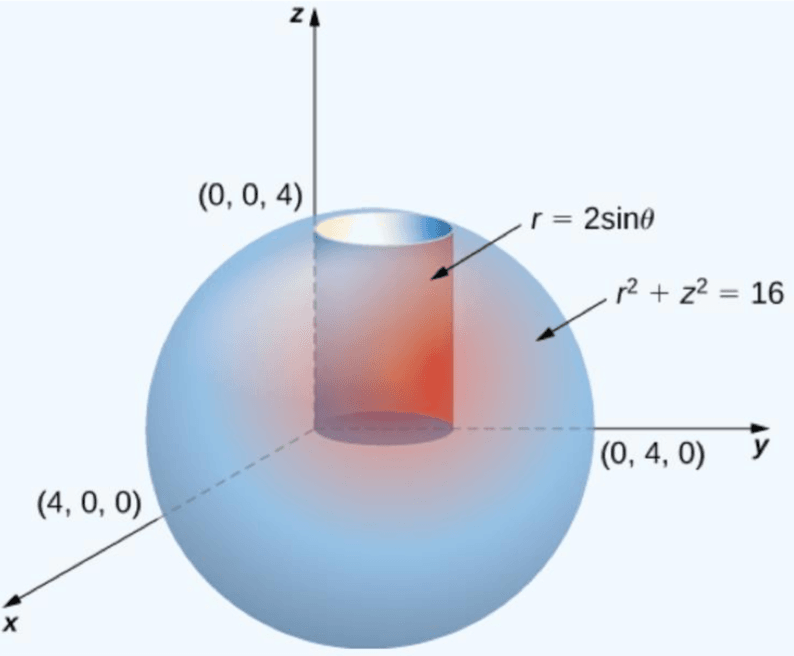 Solution
SolutionConsider the region inside the right circular cylinder with equation , bounded below by the -plane and bounded above by the sphere with radius 4 centered at the origin. Set up a triple integral over this region for a function in cylindrical coordinates.
The triple integral in cylindrical coordinates for a function is given by:
We need to find the bounds for , , and that describe the region .
The region is bounded below by the -plane, which corresponds to the plane . The region is bounded above by the sphere of radius 4 centered at the origin. The equation of this sphere in Cartesian coordinates is . In cylindrical coordinates, since , this becomes .
Solving for , we get (we take the positive root for the upper hemisphere). Therefore, the limits for are:
The projection of the volume onto the -plane (the xy-plane) is determined by the cylinder . For any given angle , the radius extends from the origin () to the edge of the cylinder (). Therefore, the limits for are:
To find the limits for , we analyze the base of the cylinder in the xy-plane. To ensure the radius is non-negative, we must have . This condition is met for angles in the first and second quadrants.
The curve starts at the origin when (since ), traces a full circle, and returns to the origin when (since ). Therefore, the limits for are:
Combining these limits, the triple integral is set up as follows:
Find the volume of solid bound by and
SolutionWe want to find the volume of the solid region bounded above by the plane and bounded below by the cone .
The equations involve , and the solid has an axis of symmetry along the z-axis. This makes cylindrical coordinates the most convenient choice. The coordinate transformations are:
The volume element in cylindrical coordinates is . The equations of the bounding surfaces become:
- Cone:
- Plane:
The volume is given by the triple integral over the region E:
We need to determine the limits of integration for , , and .
Limits for : The solid is bounded below by the cone () and above by the plane (). Thus, the limits for are:
Limits for and : The limits for and are determined by the projection of the solid onto the xy-plane. This projection is the region where the cone intersects the plane. We find this by setting the z-values equal:
This describes a circle of radius 2 centered at the origin. Therefore, the radius ranges from to , and the angle ranges over a full circle from to .
The Integral: Combining these limits, the volume integral is:
We evaluate the integral from the inside out.
Step 1: Integrate with respect to
Step 2: Integrate with respect to
Substitute the result from Step 1 into the next integral:
Step 3: Integrate with respect to
Substitute the result from Step 2 into the final integral:
The volume of the solid bounded by the plane $z=2$ and the cone $z=\sqrt{x^2+y^2}$ is:
$$
V = \frac{8\pi}{3}
$$
:::
Use spherical coordinates to find the volume of the region outside the sphere and inside the sphere with .
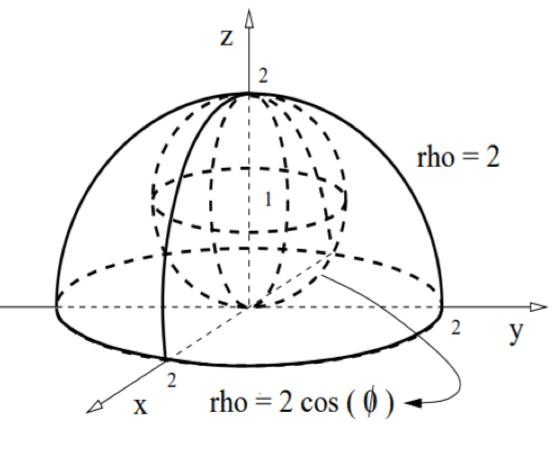 Solution
SolutionIntroduce the substitution for the integral: , .
When , .
When , .
Now substitute into the integral:
So, the entire expression for becomes:
Given a solid bound by and , find the mass density if the mass density is directly proportional to the square of the distance from origin.
SolutionThe problem states that the mass density, let's call it , is directly proportional to the square of the distance from the origin. The square of the distance from the origin to a point is .
Therefore, the density function is:
where is the constant of proportionality.
Given the geometry of the solid (bounded by a cone), it is best to use spherical coordinates . In spherical coordinates, the square of the distance from the origin is simply . So, the density function becomes:
The solid is bounded by the plane and the cone . We must convert these boundaries into spherical coordinates.
The Cone: In cylindrical coordinates, the cone is . Using the transformations and , we get:
This means the cone forms a constant angle with the positive z-axis. The solid is above this cone, so the angle ranges from the z-axis () to the cone itself.
The Plane: The upper bound is the plane . Using :
The distance starts from the origin () and extends to this plane.
The Angle : Since the solid is symmetric about the z-axis, the angle completes a full revolution.
The total mass is the triple integral of the density function over the volume of the solid. The volume element in spherical coordinates is .
Step 1: Integrate with respect to
Step 2: Integrate with respect to
We use the substitution , so . We rewrite .
The limits change: when ; when .
Step 3: Integrate with respect to
The total mass of the solid is:
where is the constant of proportionality.
Find the mass of ,, where is region bound by and .
SolutionWe need to find the mass of a solid region . The density is given by the function . The solid is bounded by the surfaces:
- A paraboloid opening along the y-axis:
- A plane perpendicular to the y-axis:
The formula for mass is given by the triple integral of the density function over the region :
The solid has a circular cross-section in the xz-plane and is symmetric about the y-axis. This suggests using a modified version of cylindrical coordinates. Let's define our coordinates as follows:
In this system, . The volume element is .
The bounding surfaces and the density function become:
- Paraboloid:
- Plane:
- Density:
We set up the integral in the order .
Limits for : For any point in the xz-plane, the vertical extent of the solid goes from the paraboloid surface up to the plane.
Limits for and : The projection of the solid onto the xz-plane is a disk. The radius of this disk is found at the intersection of the two surfaces:
Thus, the radius ranges from the y-axis () to the edge of the disk (). Since the disk is complete, the angle ranges over a full circle.
Now we substitute the density, volume element, and limits into the mass formula.
We evaluate the integral from the inside out.
Step 1: Integrate with respect to
Step 2: Integrate with respect to
Step 3: Integrate with respect to
The total mass of the solid is: